When Do You Learn Pi? This fundamental mathematical constant, π, is a gateway to understanding numerous concepts across mathematics and science. Discover the exciting journey of learning pi, from its basic definition to its advanced applications, with valuable resources from LEARNS.EDU.VN, enhancing your numerical literacy and problem-solving abilities. Unlock the power of pi and explore its significance in geometry, trigonometry, calculus, and beyond.
1. The Intriguing Introduction to Pi
Pi (π), a lowercase Greek letter, is an enduring mathematical constant representing the ratio of a circle’s circumference to its diameter. No matter the size of the circle, dividing the distance around it by the distance across will always yield a value close to pi, roughly 3.14159. Pi is an irrational number, meaning its decimal representation never ends and never settles into a repeating pattern. This seemingly simple ratio unlocks a world of complex and fascinating mathematics.
1.1. Why Pi is Important
Pi’s significance extends far beyond the classroom. It’s a cornerstone of geometry, playing a crucial role in calculating the area and circumference of circles, as well as the surface area and volume of spheres, cylinders, and cones. But its influence doesn’t stop there. Pi appears in fields like trigonometry, calculus, complex analysis, statistics, and even physics. Understanding pi is essential for anyone pursuing careers in science, technology, engineering, and mathematics (STEM).
Pi is a fascinating and ubiquitous constant with a rich history and endless applications. Exploring the intricacies of pi can be an engaging and rewarding intellectual pursuit. Let’s delve deeper into understanding the concept of Pi.
1.2. A Visual Representation of Pi
Imagine a circle. The distance around its edge is called the circumference. The distance across the circle through its center is the diameter. Pi is the ratio between these two measurements.
This means if you lay the diameter of a circle along its circumference, it would take approximately 3.14 diameters to cover the entire distance. The more digits we use, the more accurately we can describe relationships between circles, spheres, and the world around us.
1.3. Common Approximations of Pi
While pi has infinite digits, it is most commonly approximated as 3.14 or the fraction (frac{22}{7}). These approximations are useful for quick calculations, but for more precise applications, it’s important to use a calculator or computer that can store and calculate with many more digits of pi.
2. The Historical Journey of Pi
The quest to understand and calculate pi has spanned millennia, involving brilliant minds from various civilizations.
2.1. Ancient Civilizations and Pi
- Babylonians (c. 1900-1680 BC): Used (frac{25}{8}) = 3.125 as an approximation of pi.
- Egyptians (c. 1650 BC): Approximated pi as ((frac{16}{9})^{2}) ≈ 3.16.
These early approximations, while not incredibly accurate, demonstrate the early recognition of the relationship between a circle’s circumference and diameter.
2.2. Archimedes: The First Algorithmic Approach
Archimedes (c. 250 B.C.E.), a Greek mathematician, developed a groundbreaking method for approximating pi. He inscribed and circumscribed polygons around a circle, increasing the number of sides to get closer and closer to the actual circumference.
By using 96-sided polygons, he proved that (frac{223}{71}) < pi < (frac{22}{7}), providing a more accurate range for the value of pi.
2.3. The Renaissance and the Symbol Pi
The Renaissance period brought significant advancements in mathematics and the study of pi. While the concept of pi had been around for centuries, it wasn’t until 1647 that English mathematician William Oughtred started using the Greek letter π to represent the ratio of a circle’s circumference to its diameter in his publication Clavis Mathematicae. It wasn’t widely adopted until Leonhard Euler popularized the symbol in 1737. The letter π was chosen because it’s the first letter of the Greek word “perimetros,” which means circumference.
2.4. Proving Pi’s Irrationality and Transcendence
- 1767: Swiss mathematician Johann Heinrich Lambert proved that pi is irrational.
- 1882: Ferdinand von Lindemann proved that pi is transcendental, meaning it cannot be a solution to a polynomial equation with rational coefficients.
Lindemann’s proof had significant implications. It demonstrated that “squaring the circle,” a long-standing mathematical problem, was impossible. Squaring the circle refers to constructing a square with the same area as a given circle using only a compass and straightedge.
2.5. Pi in the Digital Age
With the advent of modern computers, the calculation of pi has reached incredible levels of precision. As of the latest calculations, pi has been computed to over 31 trillion digits. Despite the fact that only the first 39 digits are needed to be able to perform all calculations in our observable universe with virtually no error, the pursuit of finding new digits continues and helps to test new supercomputers and algorithms.
This quest is driven not only by the pursuit of mathematical accuracy but also by the desire to understand the nature of pi and to search for any patterns or rules within its infinite sequence of digits.
2.6. Embracing the Legacy of Pi with LEARNS.EDU.VN
The history of pi is a testament to human curiosity and the power of mathematical exploration. At LEARNS.EDU.VN, we encourage you to delve into this rich history and discover the fascinating stories behind this fundamental constant.
3. Where and When Do You Learn Pi in the Curriculum?
Pi is not confined to a single grade level. It’s a concept that is introduced and revisited throughout a student’s mathematical journey, gradually increasing in complexity and application.
3.1. Middle School: Introducing Pi
This is when most students are formally introduced to pi and its relationship to circles.
-
Circumference of a Circle: Students learn that the circumference (C) of a circle is calculated using the formula C = πd (where d is the diameter) or C = 2πr (where r is the radius).
-
Area of a Circle: Students learn that the area (A) of a circle is calculated using the formula A = πr².
At this stage, students primarily use pi in practical calculations involving circles.
3.2. High School: Expanding the Application of Pi
As students progress to high school, they encounter pi in more advanced geometric and trigonometric contexts.
-
3D Shapes:
-
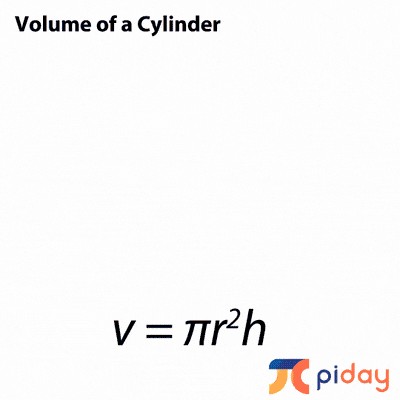
Cylinders: Students learn to calculate the surface area (2πr² + h(2πr)) and volume (πr²h) of cylinders.
-
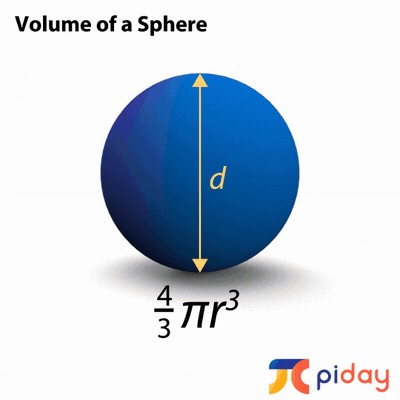
Spheres: Students learn to calculate the surface area (4πr²) and volume ((frac{4}{3})πr³) of spheres.
-
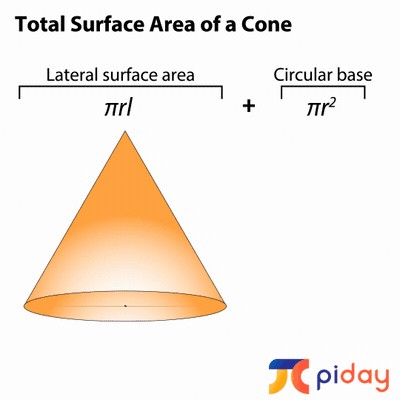
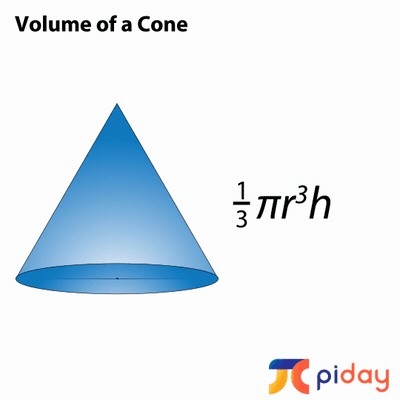
Cones: Students learn to calculate the surface area (πrl + πr²) and volume ((frac{1}{3})πr²h) of cones.
-
-
Radians: Angles can be measured in both degrees and radians. A radian is defined as an arc that has the same measure as the radius of a circle. Since π diameters equal circumference, 2π radius lengths also equals circumference. Therefore, 360 degrees is the same as 2π radians, 180 degrees equals π radians, 90 degrees equals (frac{pi}{2}) radians, etc.
-
Arcs: Students learn that the length of an arc created by a central angle θ is a fraction of the circumference of a circle: arc length = θr.
-
Sectors: The area of a sector created by a central angle θ is a fraction of the area of a circle: area of sector = (theta frac{r^{2}}{2}).
3.3. Calculus: Pi and Advanced Applications
Calculus takes the understanding of pi to a new level.
-
Calculating Volumes of Solids: Students learn how to calculate the volume of solids formed by rotating 2-dimensional surfaces around different axes using methods like the disk method, washer method, and shell method.
-
Trigonometric Functions: Pi is fundamental to understanding trigonometric functions like sine, cosine, and tangent, which are essential for modeling periodic phenomena.
-
Infinite Series: Pi appears in various infinite series, such as the Leibniz formula for π: (frac{pi}{4} = 1 – frac{1}{3} + frac{1}{5} – frac{1}{7} + …)
3.4. The Ever-Expanding Role of Pi
The journey of learning pi doesn’t end with formal education. As you delve deeper into mathematics, science, and engineering, you’ll continue to discover new and fascinating applications of this fundamental constant.
4. Beyond the Classroom: Real-World Applications of Pi
Pi is more than just a number in a textbook. It’s a fundamental constant that appears in numerous real-world applications, impacting our daily lives in ways we may not even realize.
4.1. Engineering and Construction
- Architecture: Pi is crucial for designing and constructing circular structures, arches, and domes. Architects and engineers use pi to calculate the dimensions, surface areas, and volumes of these structures, ensuring their stability and aesthetic appeal.
- Civil Engineering: Pi is essential for calculating the length of curved roads, the volume of cylindrical tanks, and the design of circular pipes and tunnels. Civil engineers rely on accurate calculations involving pi to ensure the safety and efficiency of infrastructure projects.
4.2. Physics
- Wave Motion: Pi appears in equations describing wave phenomena, such as sound waves, light waves, and water waves. Physicists use pi to calculate the wavelength, frequency, and amplitude of waves, helping them understand and predict their behavior.
- Electromagnetism: Pi is used in calculating the magnetic field produced by a current-carrying wire and the capacitance of a cylindrical capacitor. Electrical engineers and physicists use these calculations to design and analyze electrical circuits and devices.
- Quantum Mechanics: Pi appears in the Schrödinger equation, a fundamental equation in quantum mechanics that describes the behavior of subatomic particles. Pi helps physicists understand the probabilities and uncertainties associated with quantum phenomena.
4.3. Computer Science
- Cryptography: Pi can be used to generate random numbers for cryptographic algorithms. Cryptographers rely on the unpredictable nature of pi to create secure encryption keys.
- Data Compression: Pi can be used in algorithms for compressing data, allowing for more efficient storage and transmission of information.
4.4. Navigation
- GPS Systems: Pi is used in calculating the distance between two points on the Earth’s surface, which is essential for GPS navigation systems.
- Air and Sea Travel: Pi is used in calculating flight paths and shipping routes, ensuring efficient and safe transportation.
4.5. Art and Design
- Creating Circular Designs: Artists and designers use pi to create perfectly circular designs in various mediums, from paintings and sculptures to graphic design and fashion.
4.6. The Enduring Relevance of Pi
These are just a few examples of how pi is used in the real world. As technology advances and new discoveries are made, the applications of pi will continue to expand, highlighting its enduring relevance in our lives.
5. Tips and Tricks for Learning and Remembering Pi
Pi may seem daunting with its infinite digits, but there are strategies to make learning about it more accessible and enjoyable.
5.1. Mnemonic Devices
One popular technique is using mnemonic devices to remember the digits of pi. A mnemonic is a sentence or phrase where the number of letters in each word corresponds to a digit of pi. For example:
- “How I wish I could calculate pi” (3.1415)
You can find many other mnemonic devices online or create your own!
5.2. Visual Aids
Visual aids can also be helpful. Drawing circles and illustrating the relationship between circumference and diameter can solidify your understanding of what pi represents.
5.3. Real-World Examples
Connecting pi to real-world examples, like calculating the circumference of a pizza or the area of a circular garden, can make the concept more relatable and memorable.
5.4. Practice Problems
The best way to master pi is to practice using it in various calculations. Work through example problems in textbooks or online resources.
5.5. Explore the History of Pi
Learning about the history of pi and the mathematicians who dedicated their lives to understanding it can make the subject more engaging and inspiring.
5.6. Celebrate Pi Day
Join the global celebration of Pi Day on March 14th (3/14)! Participate in activities, games, and discussions related to pi to reinforce your understanding and appreciation of this fundamental constant.
5.7. Resources at LEARNS.EDU.VN
LEARNS.EDU.VN offers a variety of resources to help you learn about pi, including articles, tutorials, practice problems, and interactive simulations.
6. Frequently Asked Questions (FAQs) About Pi
Let’s address some common questions about pi to further clarify its significance and applications.
| Question | Answer |
|---|---|
| What is the value of pi? | Pi is approximately 3.14159, but its decimal representation continues infinitely without repeating. |
| Why is pi an irrational number? | Pi is irrational because it cannot be expressed as a simple fraction (frac{a}{b}), where a and b are integers. Its decimal representation is non-terminating and non-repeating. |
| How many digits of pi have been calculated? | Pi has been calculated to over 31 trillion digits using supercomputers. |
| Is there a pattern in the digits of pi? | No known repeating pattern has been found in the digits of pi. It is believed to be a normal number, meaning that all digits and sequences of digits appear with equal frequency. |
| What is Pi Day? | Pi Day is celebrated on March 14th (3/14) each year to commemorate the mathematical constant π. |
| Where does pi appear in real life? | Pi appears in various fields, including geometry, trigonometry, calculus, physics, engineering, computer science, and navigation. |
| Who discovered pi? | The concept of pi has been known for thousands of years, with early approximations dating back to ancient civilizations like the Babylonians and Egyptians. Archimedes was the first to use an algorithmic approach to calculate pi. |
| What is the significance of pi in trigonometry? | Pi is fundamental to understanding trigonometric functions like sine, cosine, and tangent. It is used to define the relationships between angles and sides of triangles and to model periodic phenomena. |
| Can pi be expressed as a fraction? | No, pi cannot be expressed as a simple fraction. It is an irrational number. The fraction (frac{22}{7}) is a common approximation of pi, but it is not its exact value. |
| Why is pi important? | Pi is essential for calculating the circumference and area of circles, the surface area and volume of spheres, cylinders, and cones, and for understanding various concepts in mathematics, science, and engineering. It is a fundamental constant with widespread applications. |



7. Elevate Your Pi Knowledge with LEARNS.EDU.VN
At LEARNS.EDU.VN, we are dedicated to providing high-quality educational resources that empower you to explore the fascinating world of mathematics. Our platform offers:
- Comprehensive Articles and Tutorials: Delve into detailed explanations of pi and its applications, written by experienced educators.
- Engaging Practice Problems: Sharpen your skills with a wide range of practice problems, from basic calculations to advanced applications.
- Interactive Simulations: Visualize the concepts of pi and its relationship to circles, spheres, and other geometric shapes.
- Personalized Learning Paths: Tailor your learning experience to your individual needs and interests.
- Expert Support: Connect with our team of expert educators for guidance and support.
7.1. Unlock Your Mathematical Potential
Whether you’re a student looking to improve your grades, a professional seeking to enhance your skills, or simply a curious individual eager to learn, LEARNS.EDU.VN has something for you.
7.2. Embark on a Journey of Discovery
Explore the captivating world of pi and discover its hidden depths. With LEARNS.EDU.VN as your guide, you’ll gain a deeper appreciation for this fundamental constant and its profound impact on our understanding of the universe.
Ready to unlock the power of pi? Visit LEARNS.EDU.VN today to explore our comprehensive resources and embark on a journey of mathematical discovery.
Contact us:
- Address: 123 Education Way, Learnville, CA 90210, United States
- WhatsApp: +1 555-555-1212
- Website: LEARNS.EDU.VN
Let learns.edu.vn be your trusted partner in learning and achieving your educational goals.